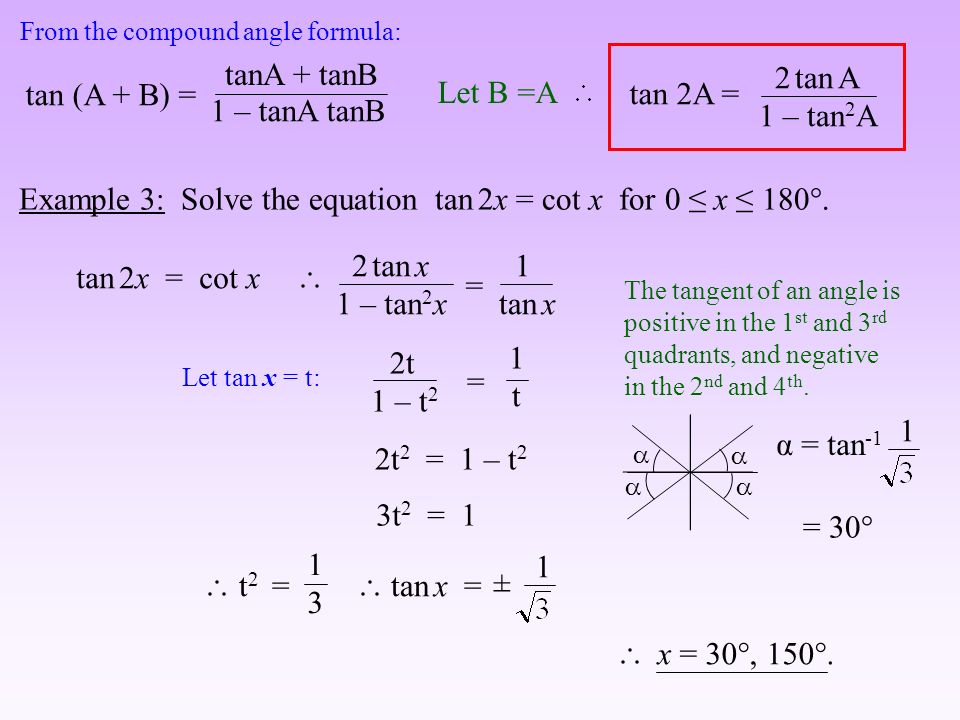
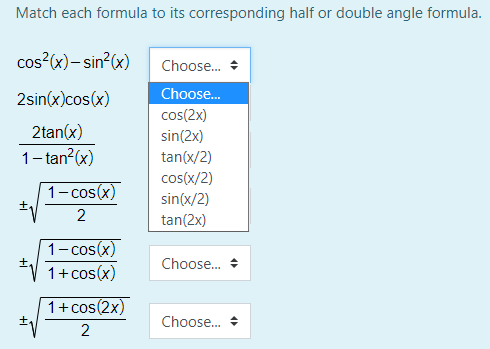
Tan2x Formula In trigonometry, Tan2x is a double angle identity Because the tangent function is a ratio of the sine and cosine functions, it may also be represented as tan2x = sin 2x/cos 2x It's an important trigonometric identity that's utilized to solve a wide range of trigonometric and integration problems
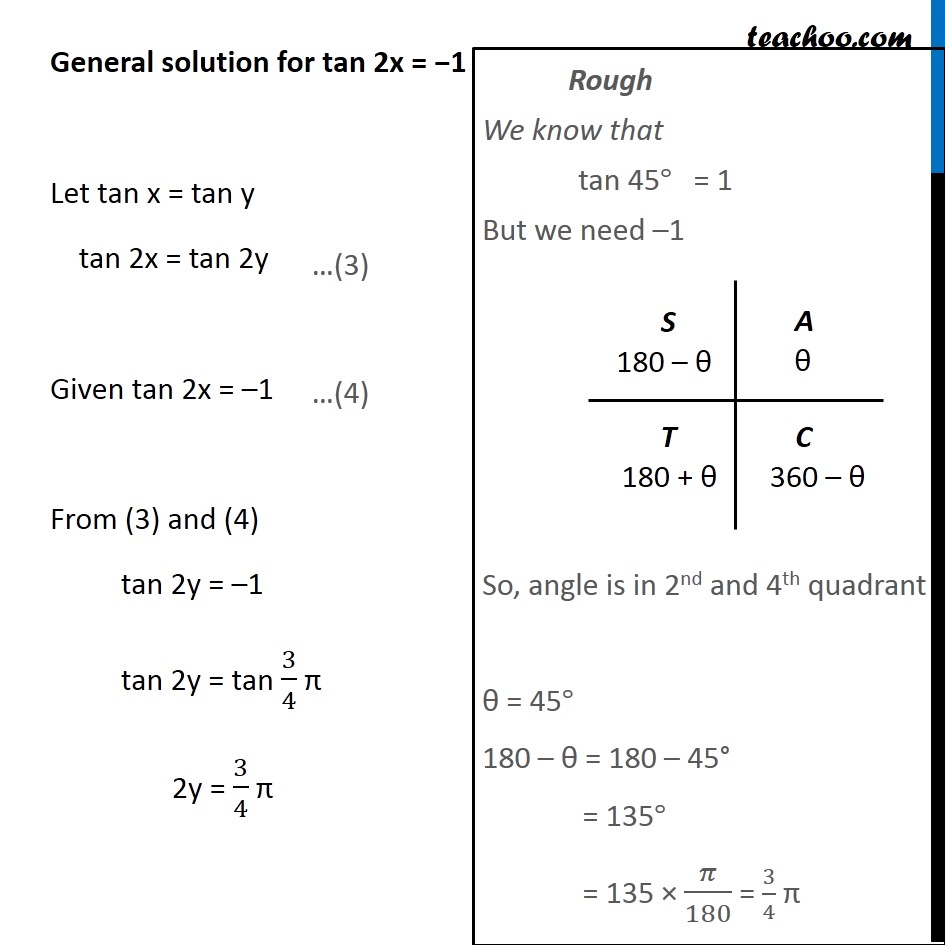
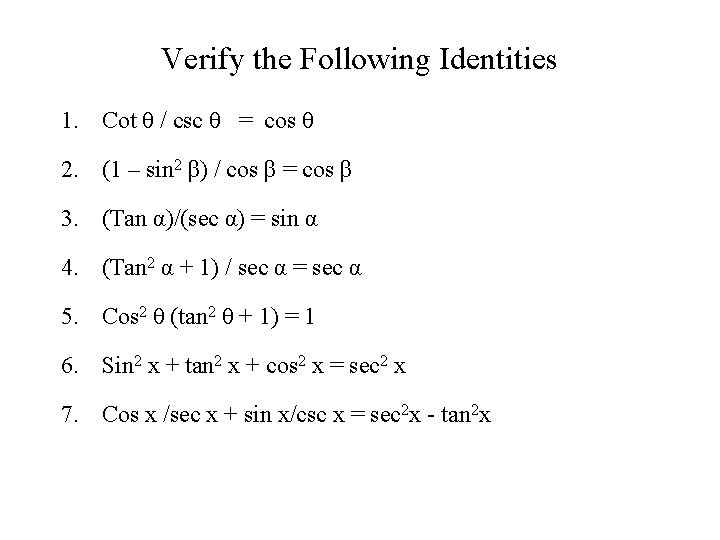
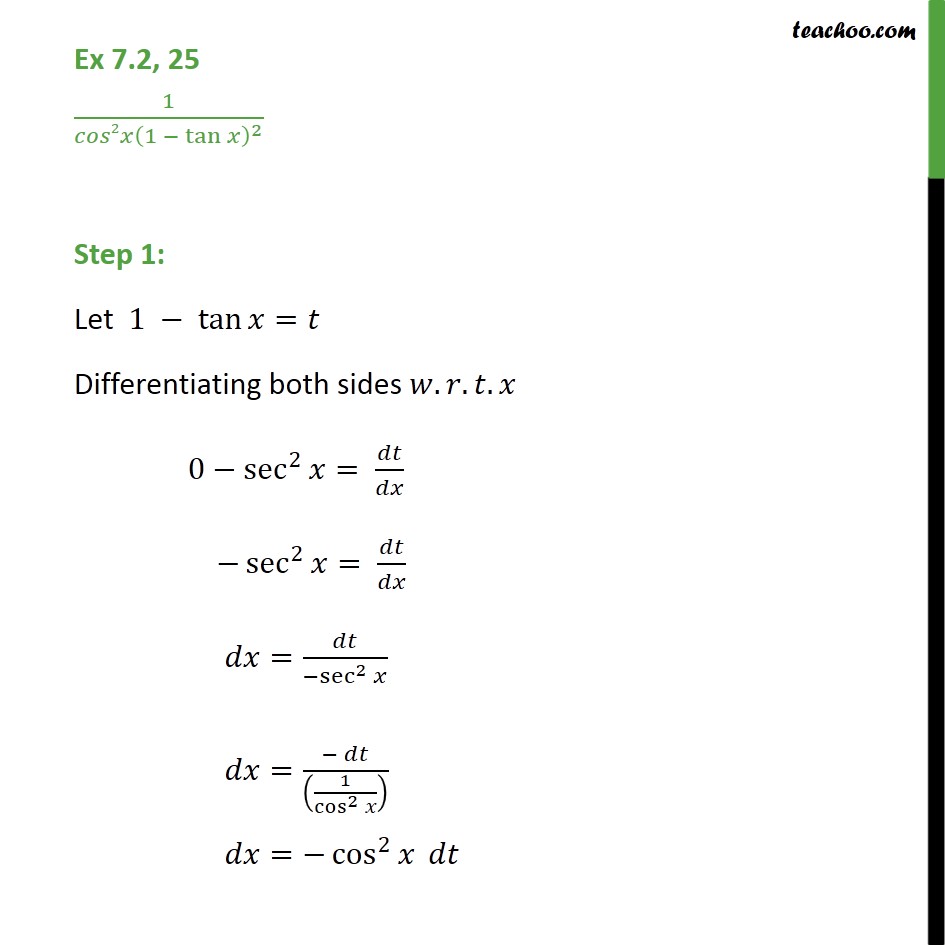
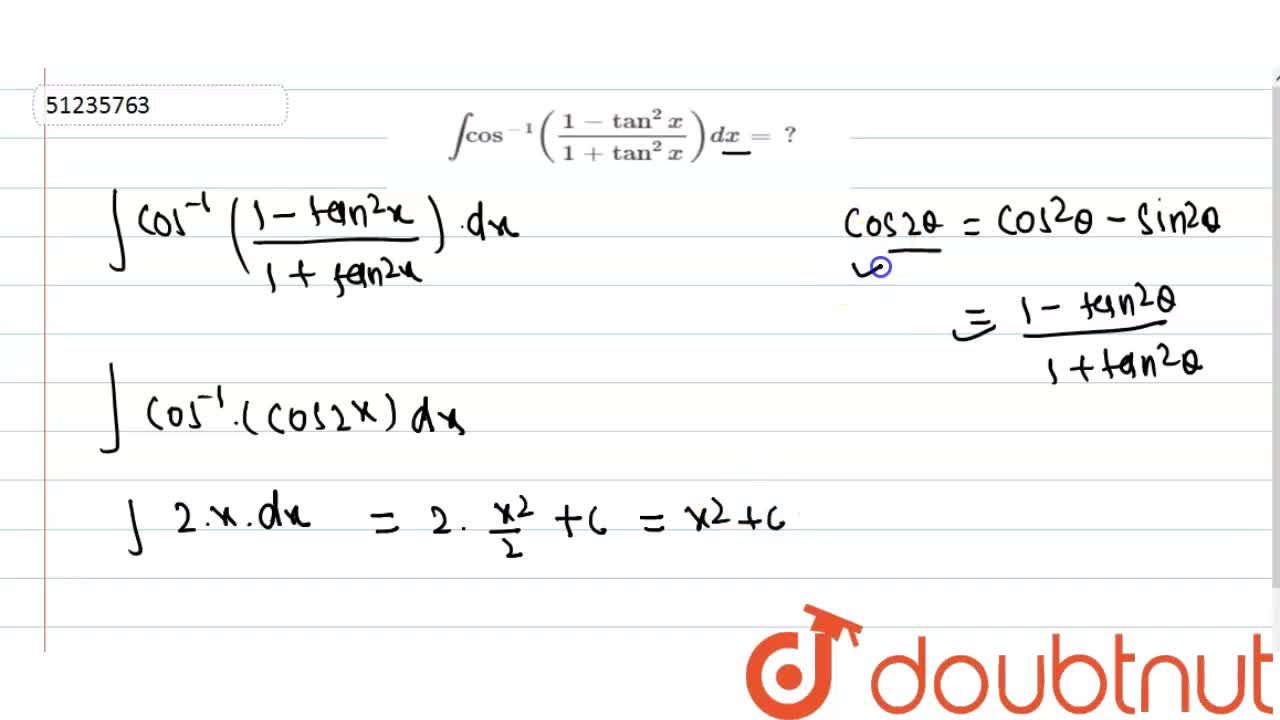
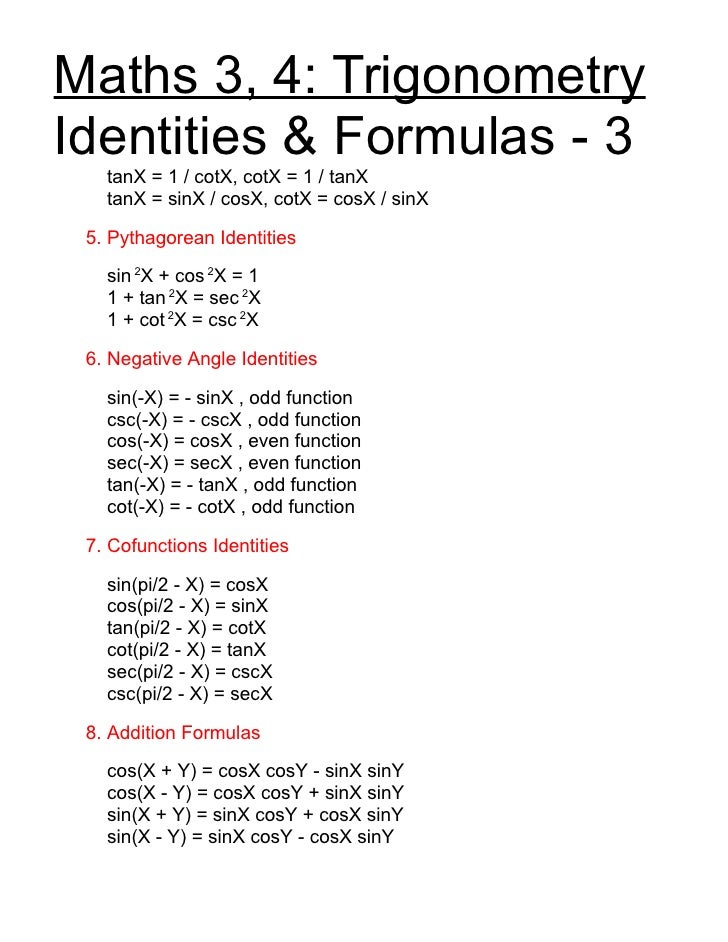
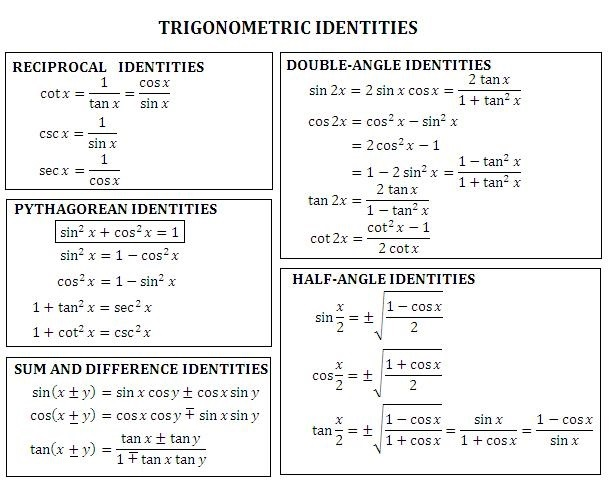
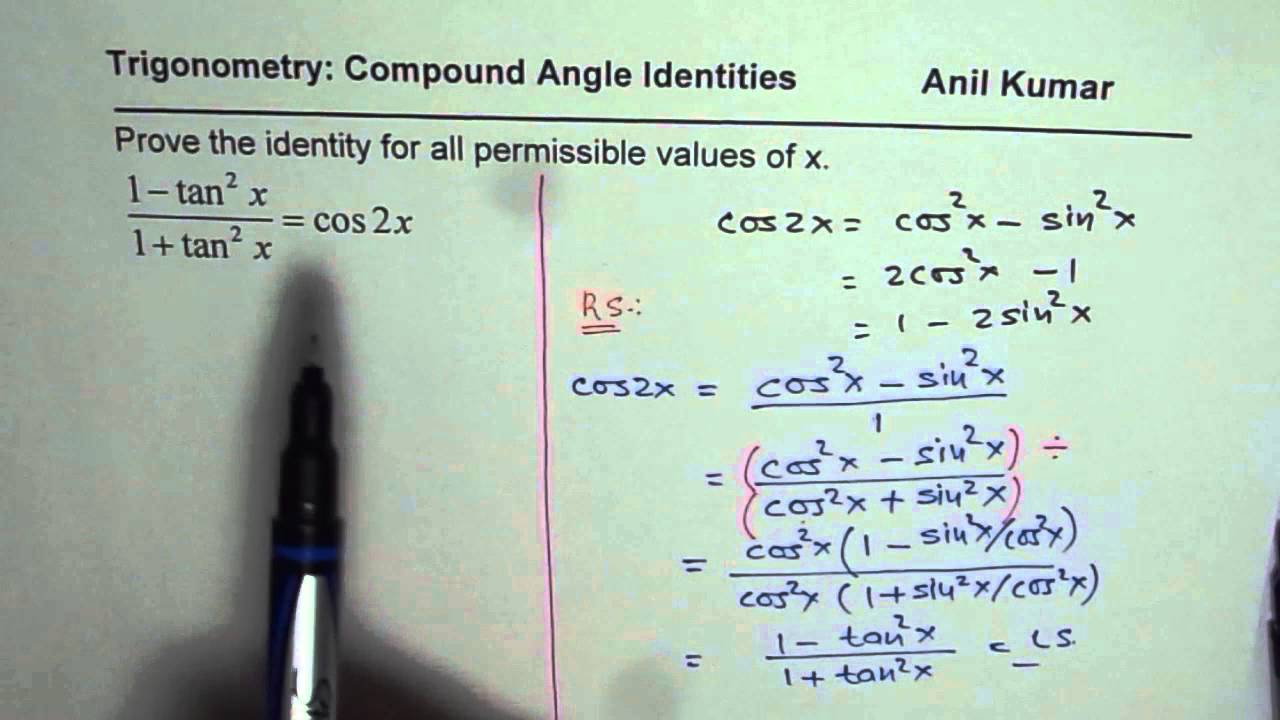
1-tan^2x identity-Cot θ = 1/tan θ sin θ = 1/cosec θ cos θ = 1/sec θ tan θ = 1/cot θ All these are taken from a rightangled triangle When the height and base side of the right triangle are known, we can find out the sine, cosine, tangent, secant, cosecant, and cotangent values using trigonometric formulasLimit for x → π for the trigonometric function (1 cosx)/tan2x HINT We have that tan2x1cosx = sin2xcos2x(1cosx) = 1−cos2xcos2x(1cosx) = 1−cosxcos2x which is not an indeterminate form How do you use the double angle formula to verify tan2x = 1cos2x1−cos2x ?
1-tan^2x identityのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 | ||
 |  |  |
 |  |  |
 | ||
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  |  |
 |  |  |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
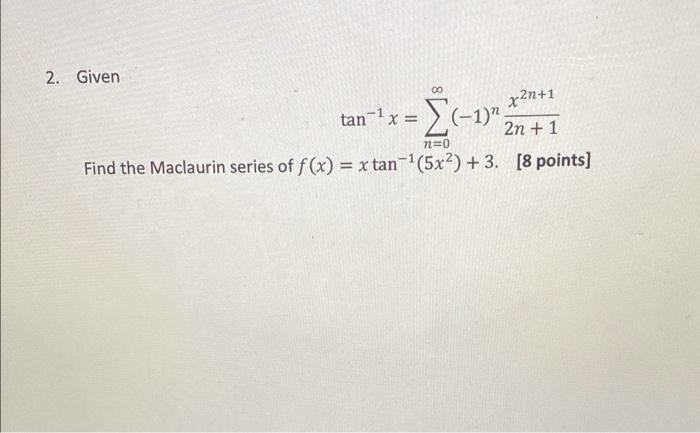 | ||
 |  |  |
 | 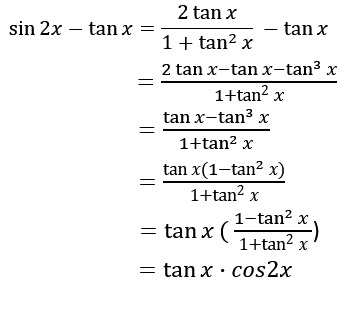 | |
 |  |  |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  |  |
 |  |  |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | |
 |  | |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 | 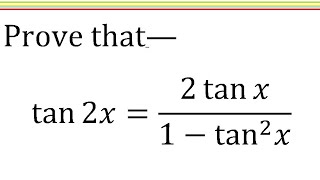 | 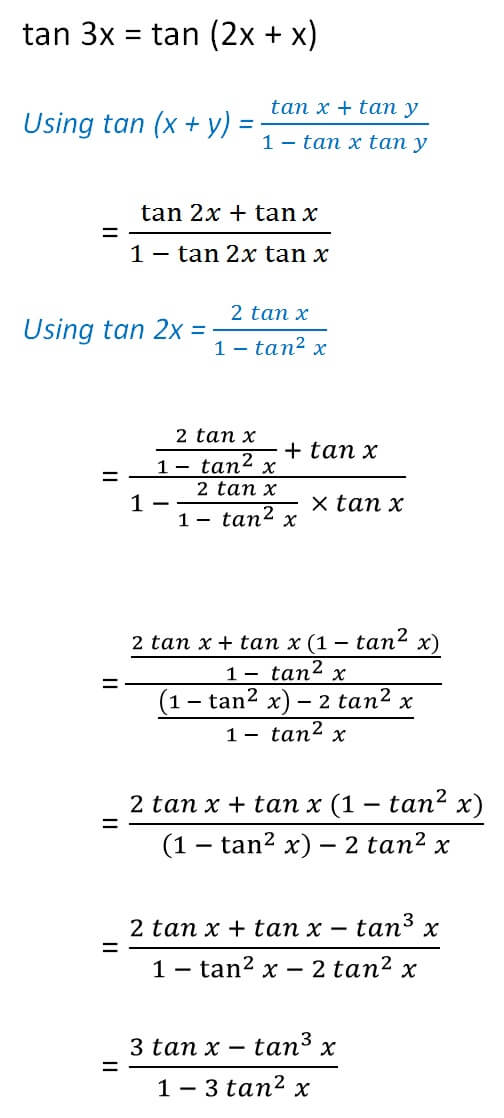 |
 |  | |
 |  | |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 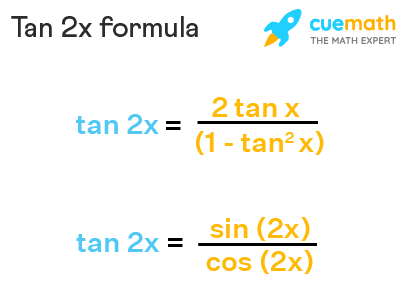 |  |
 |  |  |
 |  | |
 |  |  |
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | 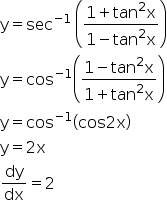 |
 | ||
「1-tan^2x identity」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
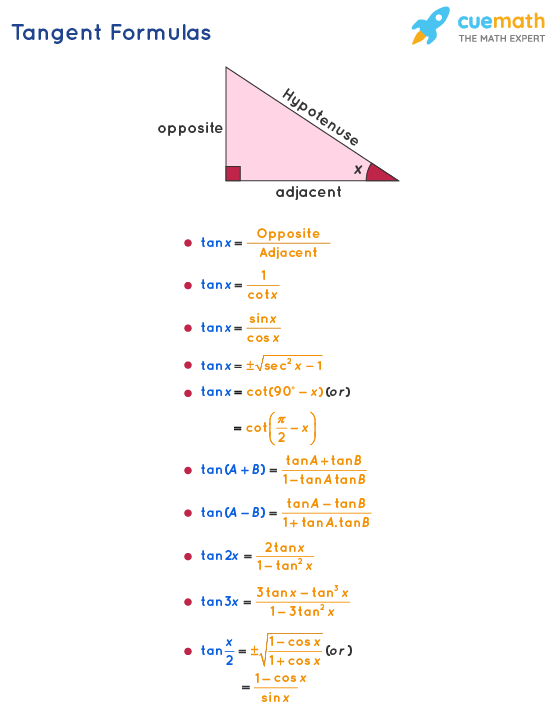 |  | |
 |  |
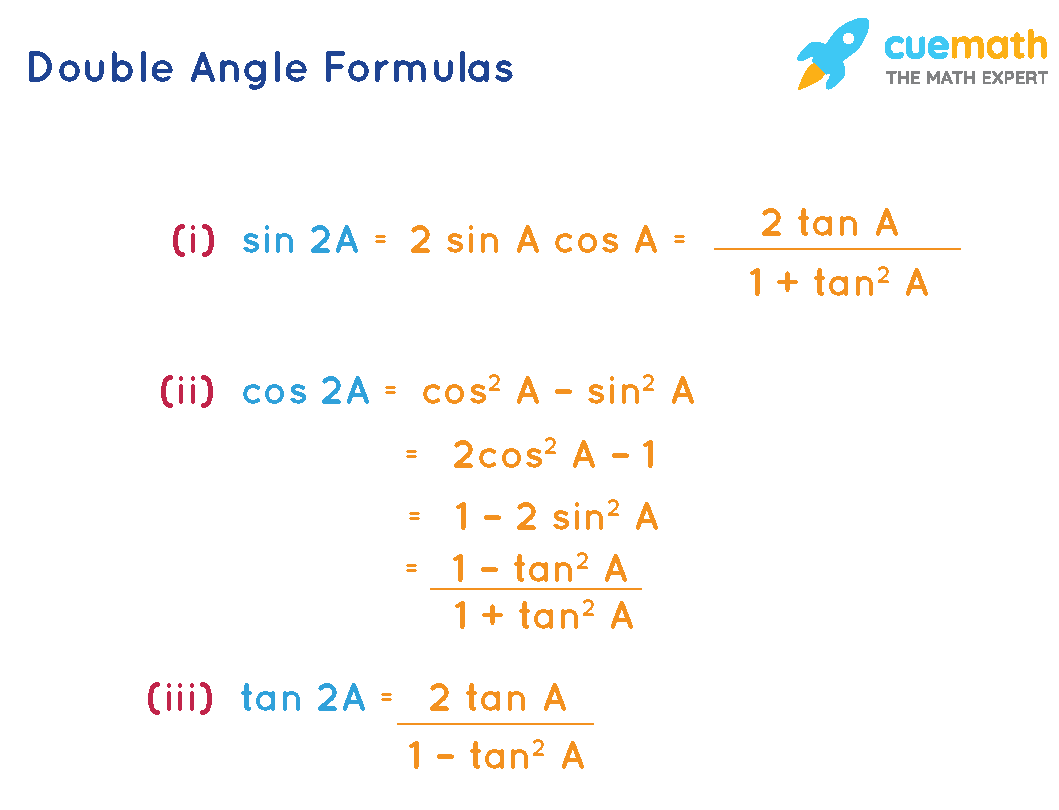
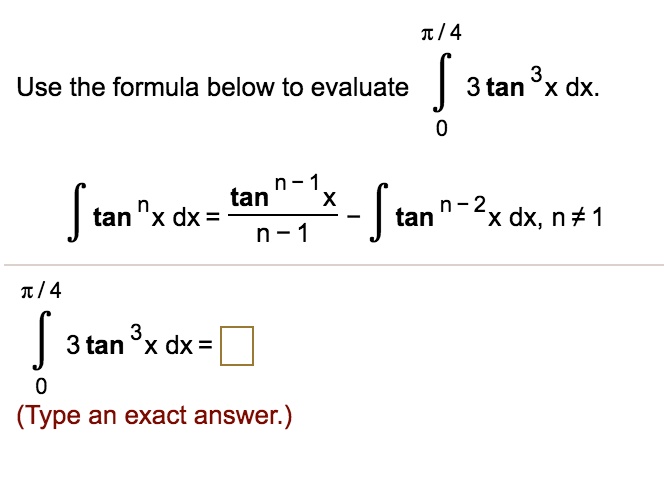
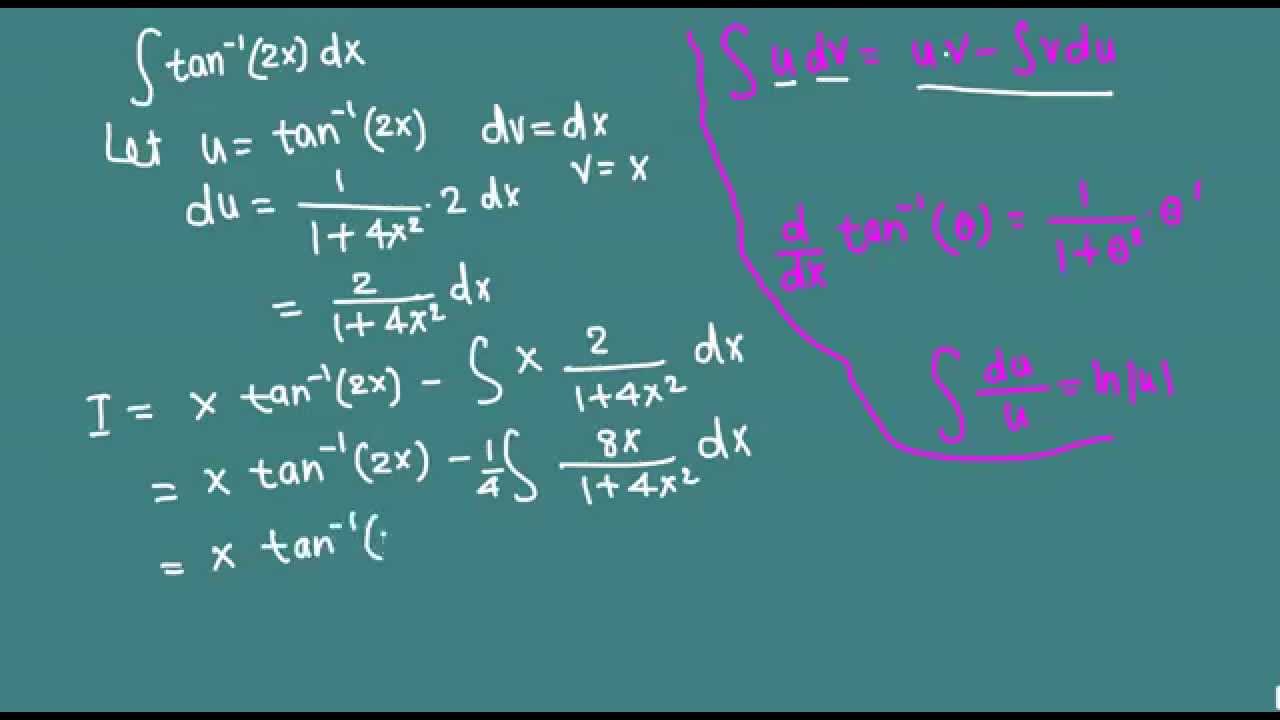
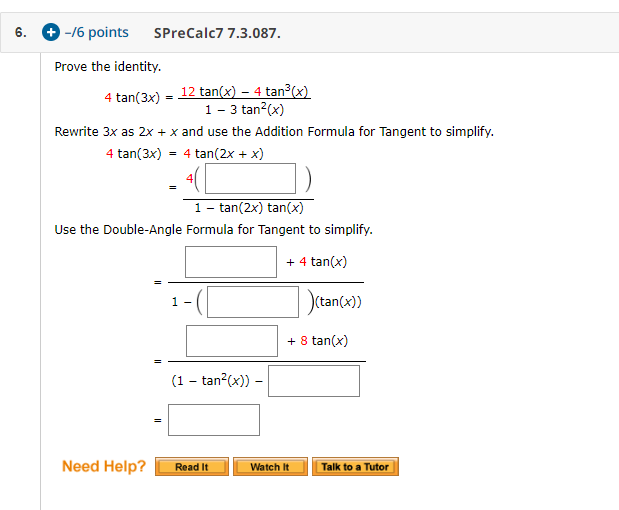
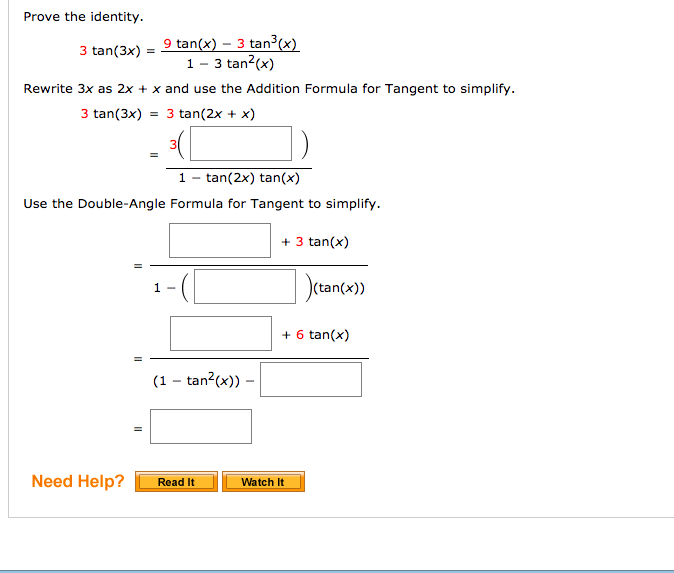
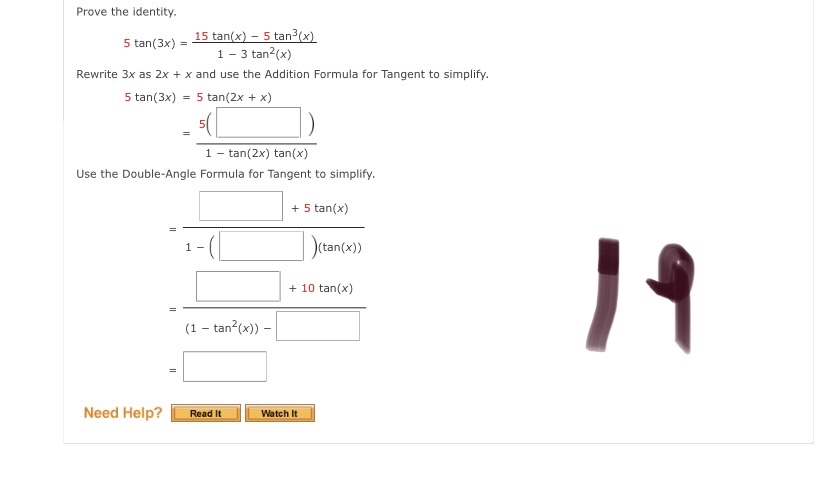
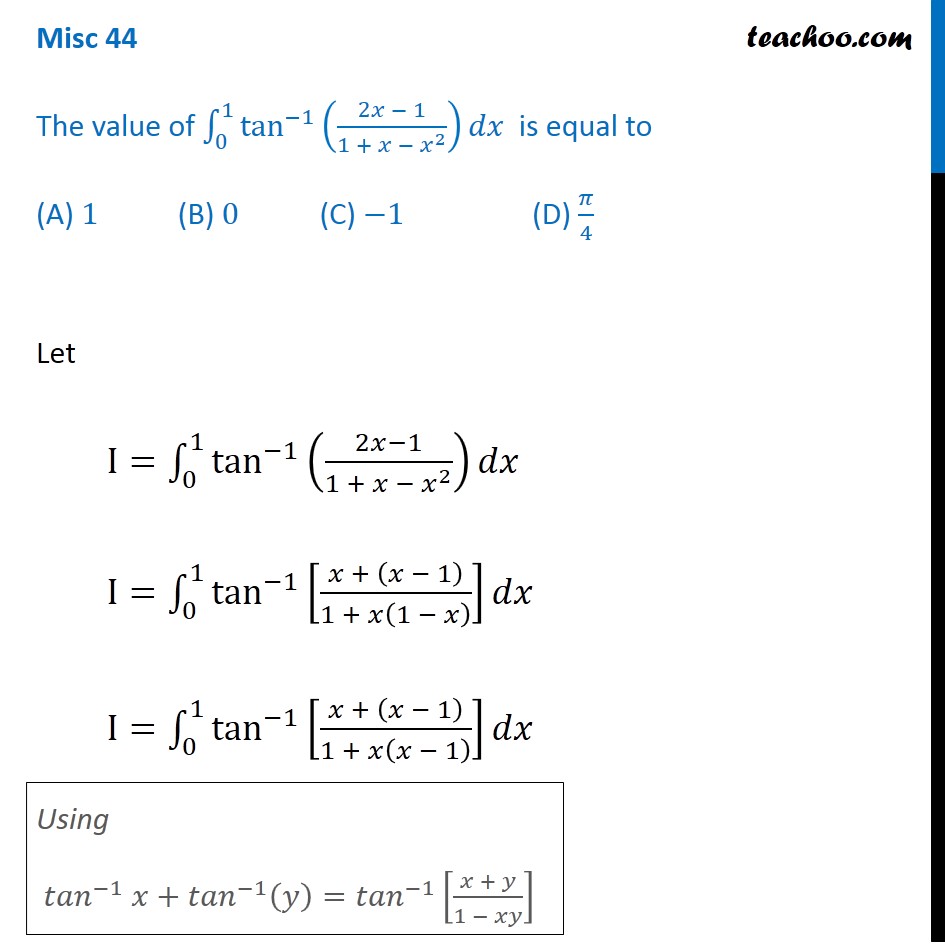
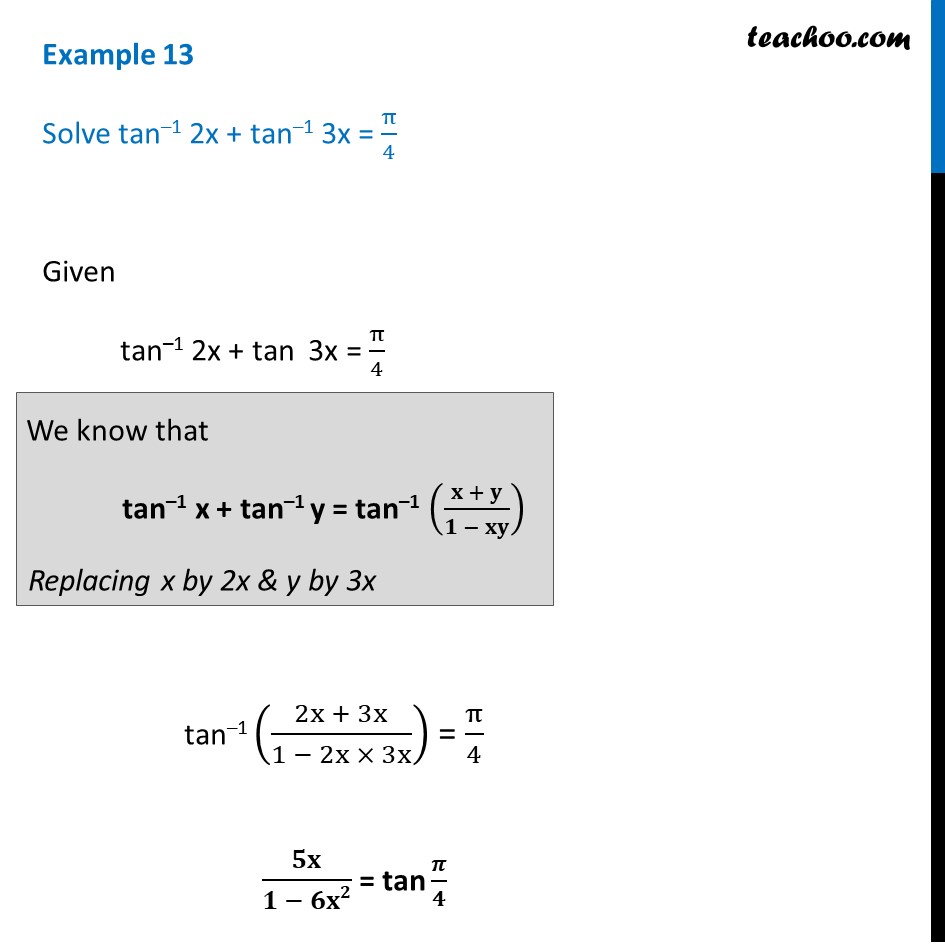
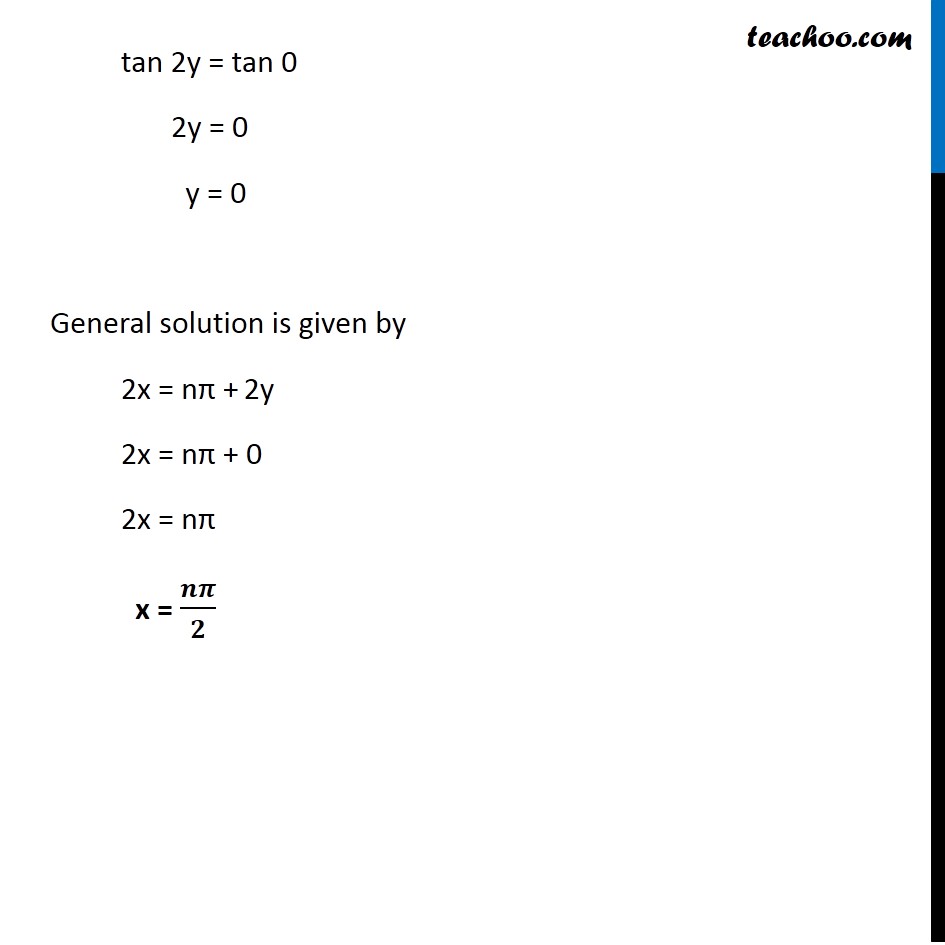
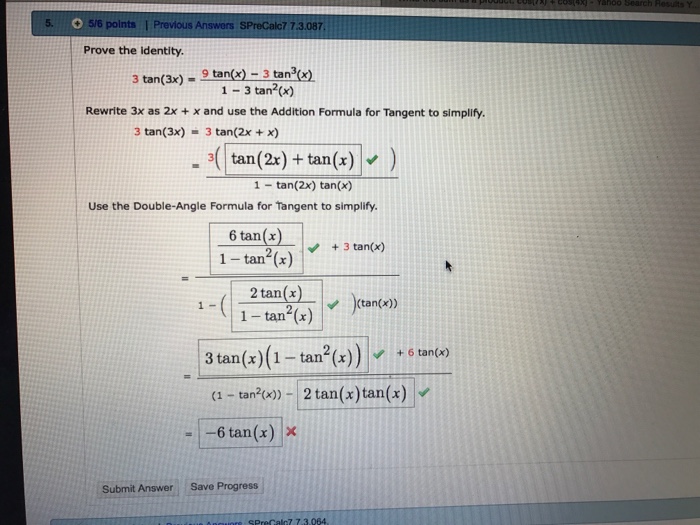
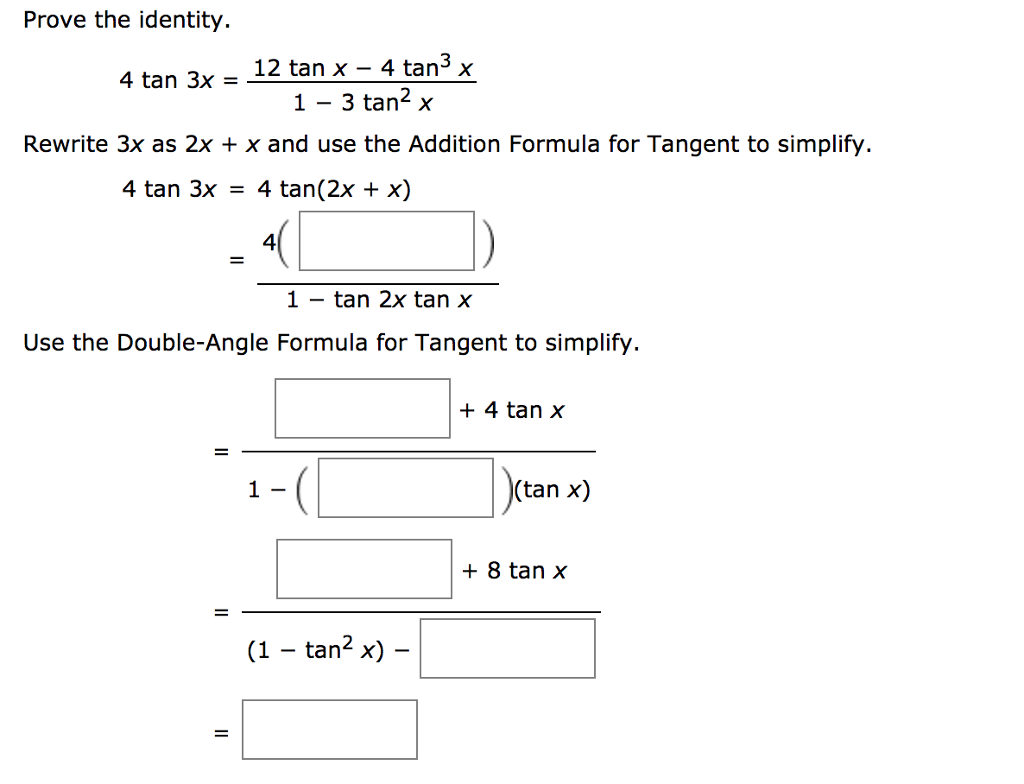
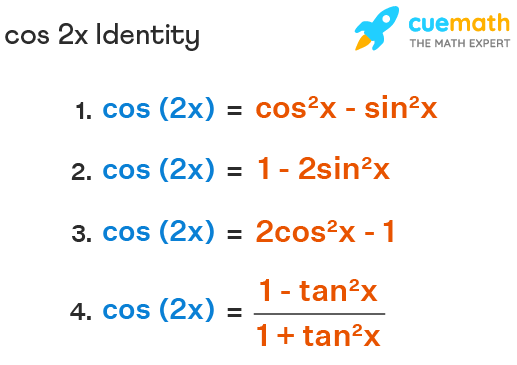
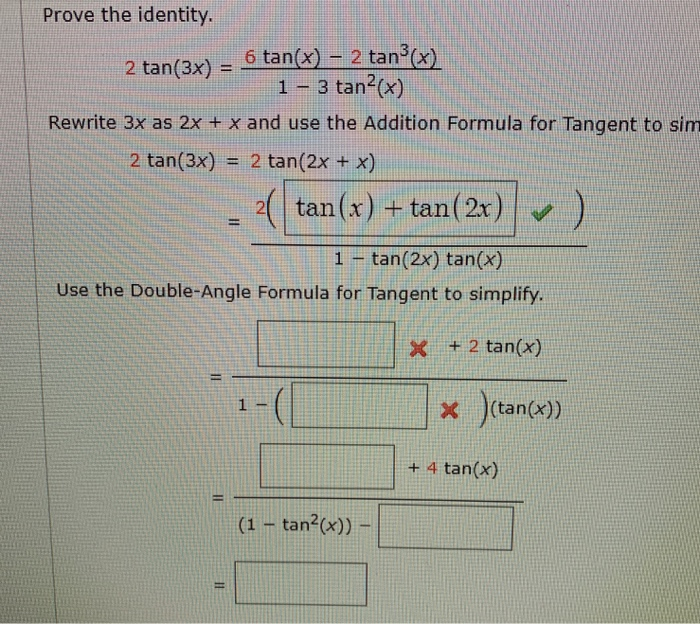
The double angle formula of tan is tan 2x = (2 tan x) / (1 tan 2 x) Tangent Formula of Triple Angle We have triple angle formulas for all trigonometric functions Among them, the triple angle formula of the tangent function is, tan 3x = (3 tan x tan 3 x) / (1The Trigonometric Formula Of Cos2x Now if you are wondering what the formula of cos2x is, let me tell you that we have five cos x formulas The trigonometric formula of cos2x = Cos²x Sin²x The trigonometric formula of c o s 2 x = 1 − 2 S i n 2 x The trigonometric formula of c
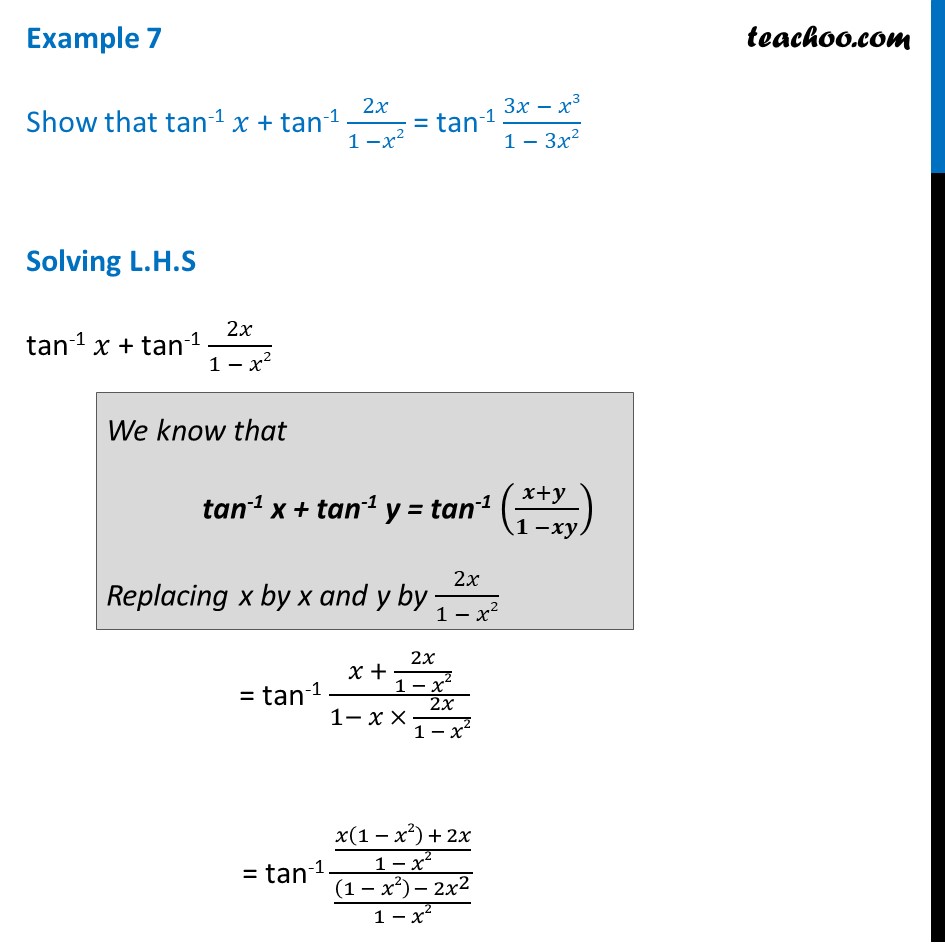
Incoming Term: 1+tan^2x formula, 1-tan^2x identity, 2tanx/1+tan^2x formula, tan^-1(2x/1-x^2) formula,




0 件のコメント:
コメントを投稿